By the end of this section, you will be able to:
The study of the chemical behavior of gases was part of the basis of perhaps the most fundamental chemical revolution in history. French nobleman Antoine Lavoisier, widely regarded as the “father of modern chemistry,” changed chemistry from a qualitative to a quantitative science through his work with gases. He discovered the law of conservation of matter, discovered the role of oxygen in combustion reactions, determined the composition of air, explained respiration in terms of chemical reactions, and more. He was a casualty of the French Revolution, guillotined in 1794. Of his death, mathematician and astronomer Joseph-Louis Lagrange said, “It took the mob only a moment to remove his head; a century will not suffice to reproduce it.”
As described in an earlier chapter of this text, we can turn to chemical stoichiometry for answers to many of the questions that ask “How much?” We can answer the question with masses of substances or volumes of solutions. However, we can also answer this question another way: with volumes of gases. We can use the ideal gas equation to relate the pressure, volume, temperature, and number of moles of a gas. Here we will combine the ideal gas equation with other equations to find gas density and molar mass. We will deal with mixtures of different gases, and calculate amounts of substances in reactions involving gases. This section will not introduce any new material or ideas, but will provide examples of applications and ways to integrate concepts we have already discussed.
Another useful application of the ideal gas law involves the determination of molar mass. By definition, the molar mass of a substance is the ratio of its mass in grams, m, to its amount in moles, n
The ideal gas equation can be rearranged to isolate n:
and then combined with the molar mass equation to yield:
This equation can be used to derive the molar mass of a gas from measurements of its pressure, volume, temperature, and mass.
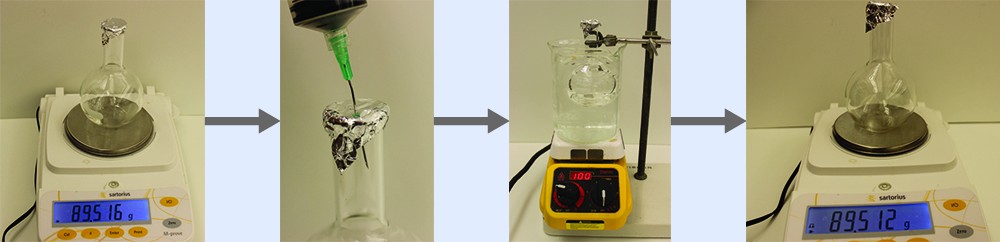
The approximate molar mass of a volatile liquid can be determined by:

Using this procedure, a sample of chloroform gas weighing 0.494 g is collected in a flask with a volume of 129 cm 3 at 99.6 °C when the atmospheric pressure is 742.1 mm Hg. What is the approximate molar mass of chloroform?
Show AnswerSince [latex]\large\mathcal=\frac[/latex] and [latex]n=\frac,[/latex] substituting and rearranging gives [latex]\mathcal=\frac,[/latex] then
A sample of phosphorus that weighs 3.243 × 10 -2 g exerts a pressure of 31.89 kPa in a 56.0 mL bulb at 550 °C. What is the molar mass of phosphorus vapor?
Show Answer 124 g/mol P4Chemical stoichiometry describes the quantitative relationships between reactants and products in chemical reactions.
We have previously measured quantities of reactants and products using masses for solids; now we can also use gas volumes to indicate quantities. If we know the volume, pressure, and temperature of a gas, we can use the ideal gas equation to calculate how many moles of the gas are present. If we know how many moles of a gas are involved, we can calculate the volume of a gas at any temperature and pressure.
What volume of hydrogen at 27 °C and 723 torr may be prepared by the reaction of 8.88 g of gallium with an excess of hydrochloric acid?
Show Answer To convert from the mass of gallium to the volume of H2(g), we need to do something like this:The first two conversions are:
Finally, we can use the ideal gas law:
Sulfur dioxide is an intermediate in the preparation of sulfuric acid. What volume of SO2 at 343 °C and 1.21 atm is produced by burning 1.00 kg of sulfur in oxygen?
Show Answer 1.30 × 10 3 LHow many grams of Zn is required in the following reaction to produce 19.6 L of hydrogen gas at 299 K and 1.07 atm?
[latex]\large \text\left(s\right)+2\text< HCl>\left(aq\right)\rightarrow 2>_\left(aq\right)+>_\left(g\right)[/latex]
Show AnswerFrom the question, we know we are looking for grams of Zn, therefore at some point we will need the molar mass of Zn (65.41 g/mol). We are given 19.6 L of H2, the pressure (1.07 atm), and temperature (299 K). We will also need a mole to mole ration between Zn and H2, since we are essentially converting units of H2 (19.6 L) to units of Zn (? g). Due to the pressure and temperature being given we will need the ideal gas law (PV = nRT). Once you realize you will be using the ideal gas law, identify how you will be using it based off the information provided. For PV = nRT, we have pressure, temperature, and volume given (we also know that R is the gas constant), therefore the only term we do not know is n (moles of gas). We now have all the pieces we need to solve the problem, so where do we start?
We start by using the ideal gas law to solve for moles of H2. Once we have moles of H2, we can then convert it to moles of Zn by using the mole to mole ratio from the chemical equation, and then to grams of Zn using the molar mass of Zn.
Use ideal gas law to determine moles of hydrogen gas:
All the units cancel except for moles, which means n = 0.853 moles H2.
Now that we know the number of moles of gas, we can use stoichiometry to find grams of Zn:
What pressure of HCl is generated if 3.44 g of Cl2 are reacted in 4.55 L at 455 K?
Show AnswerThe ideal gas law can be used to derive a number of convenient equations relating directly measured quantities to properties of interest for gaseous substances and mixtures. Appropriate rearrangement of the ideal gas equation may be made to permit the calculation of gas molar masses. The ideal gas law can also be used in relation with stoichiometry.
3. The answers are as follows:
5. The answers are as follows:
7. The answers are as follows:
From the balanced equation, we see that 2 mol of C2H6 requires 7 mol of O2 to burn completely. Gay-Lussac’s law states that gases react in simple proportions by volume. As the number of liters is proportional to the number of moles,
Licenses and Attributions CC licensed content, Shared previously